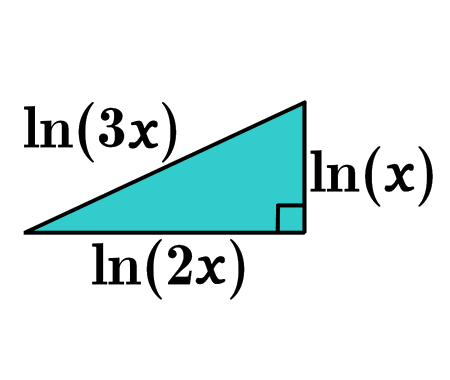Home -> Solved problems -> Find the length of the black segment

Solution

To start, let’s simplify the geometric shape following the next steps.

Step 1:

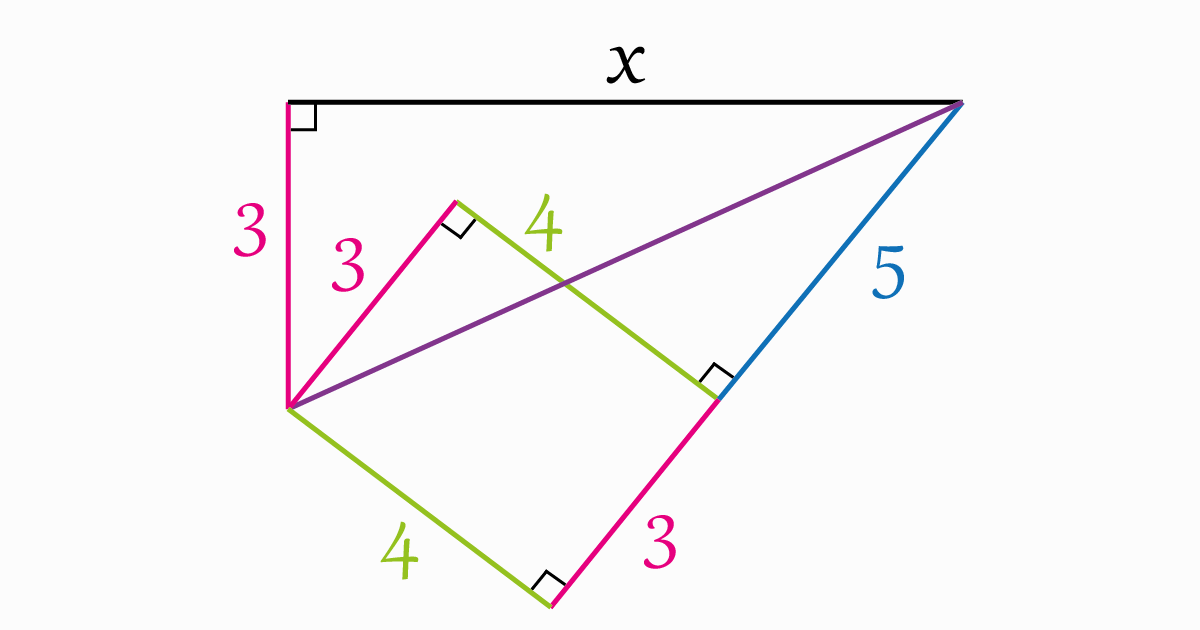
Step 2: Let \(y\) be the length of the purple segment

Now, we have a simplified version of the geometric shape. Let’s pick the right triangle in the next figure and apply Pythagoras theorem

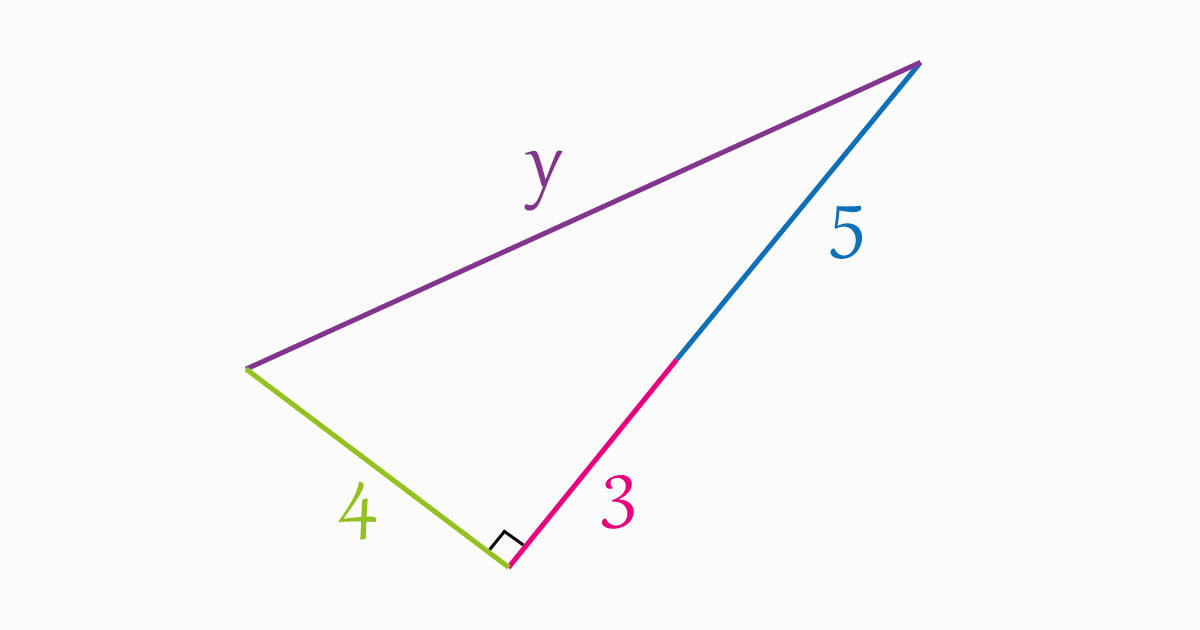
\[y^{2}=4^{2}+\left(3+5\right)^{2}\]

\[y^{2}=80\]

Same thing for the upper right triangle

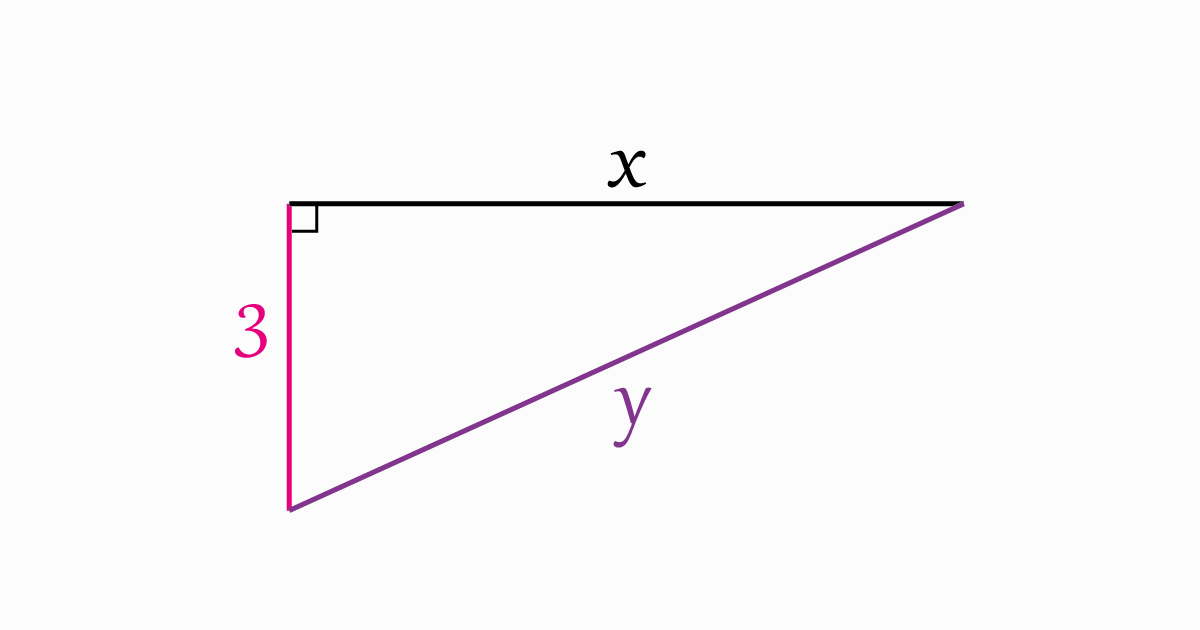
\[y^{2}=x^{2}+3^{2}\]

\[\Rightarrow x^{2}+3^{2}=80\]

\[x^{2}=71\]

\[x>0\]

\[\huge \Rightarrow x=\sqrt{71} \]

Home -> Solved problems -> Find the length of the black segment

Related Topics: Solved Problems

How far apart are the poles ?

Solution

Determine the square's side \(x\)

Solution

How Tall Is The Table ?

Solution

Find the volume of the interior of the kiln

Solution

Error to avoid that leads to:

Solution

Why 0.9999999...=1

Solution

What's the problem ?

Solution

Explain your answer without using calculator

Solution

What values of \(x\) satisfy this inequality

Solution

Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x} \) has a curvilinear asymptote \(y=x^{2}+2 x+3\)

Solution

Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?

Solution

Only one in 1000 can solve this math problem

Solution

Calculate the following

Solution

Find the limit of width and height ratio

Solution

Is \(\pi\) an irrational number ?

Solution

Solve for \(x \in \mathbb{R}\)

Solution

Prove that \(e\) is an irrational number

Solution

Prove that

Solution

Challenging problem

Solution

Solve the equation for \(x \in \mathbb{R}\)

Solution

Calculate the following limit

Solution

Calculate the following limit

Solution

Determine the angle \(x\)

Solution

Find the derivative of \(y\) with respect to \(x\)

Solution

Solve the equation for real values of \(x\)

Solution

Find the equation of the curve formed by a cable suspended between two points at the same height

Solution

Calculate the sum of areas of the three squares

Solution

Calculate the integral

Solution

Prove Wallis Product Using Integration

Solution

Calculate the radius R

Solution

Calculate the volume of Torus using cylindrical shells

Solution

Find the derivative of exponential \(x\) from first principles

Solution

Find the volume of the square pyramid as a function of \(a\) and \(H\) by slicing method.

Solution

Prove that \[\lim_{x \rightarrow 0}\frac{\sin x}{x}=1\]

Solution

Prove that

Solution

Calculate the half derivative of \(x\)

Solution

Find out what is a discriminant of a quadratic equation.

Solution

Calculate the rectangle's area

Solution

Infinitely nested radicals

Solution

Determine the square's side \(x\)

Solution

Wonderful math fact: 12542 x 11 = 137962

Explanation

Calculate the sum

Solution

What is the new distance between the two circles ?

Solution

Solve the equation for \(x\epsilon\mathbb{R}\)

Solution

Calculate the area of the Squid Game diagram blue part

Solution

Calculate the limit

Solution

Find the infinite sum

Solution

Calculate the integral

Solution

Prove that

Solution

Can we set up this tent ?

Solution

Find the value of \(h\)

Solution

Is the walk possible?

Solution

Find the length of the black segment

Solution

Home -> Solved problems -> Find the length of the black segment

Share the solution: Find the length of the black segment