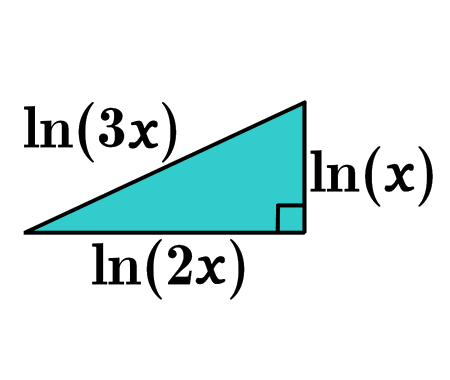Triangle problem, logarithms
Home -> Solved problems -> Triangle problem, logarithms
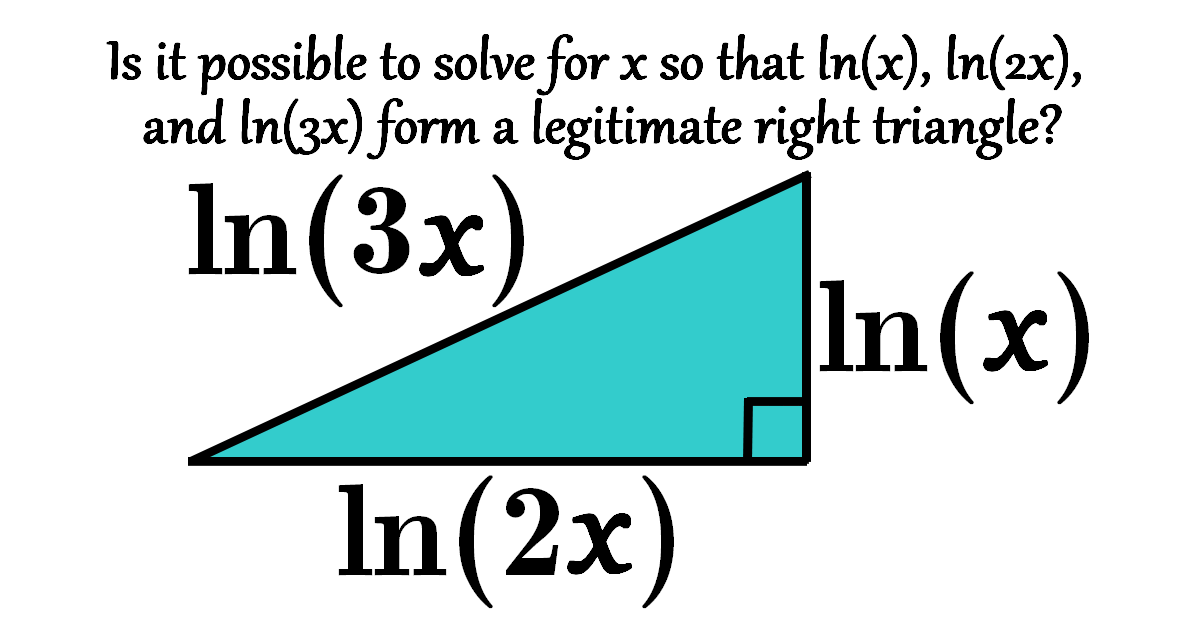
Solution
\[\begin{aligned}
&(\operatorname{Ln}(3 x))^{2}=(\operatorname{Ln}(2 x))^{2}+(\operatorname{Ln}(x))^{2} \\\\
&(\operatorname{Ln}(3)+\operatorname{Ln}(x))^{2}=(\operatorname{Ln}(2)+\operatorname{Ln}(x))^{2}+(\operatorname{Ln}(x))^{2} \\\\
&\left.(\operatorname{Ln}(3))^{2}+2 \operatorname{Ln}(3) \operatorname{Ln}(x)+(\operatorname{Ln}(x))^{2}=(\operatorname{Ln}(2))^{2}+2 \operatorname{Ln}(2) \operatorname{Ln}(x)+(\operatorname{Ln}(x))^{2}+\operatorname{Ln}(x)\right)^{2} \\\\
&(\operatorname{Ln}(3))^{2}+2 \ln (3) \operatorname{Ln}(x)-(\operatorname{Ln}(2))^{2}-2 \operatorname{Ln}(2) \operatorname{Ln}(x)-(\operatorname{Ln}(x))^{2}=0 \\\\
&(\operatorname{Ln}(x))^{2}+2(\operatorname{Ln}(2)-\operatorname{Ln}(3)) \operatorname{Ln}(x)+(\operatorname{Ln}(2))^{2}-(\operatorname{Ln}(3))^{2}=0 \\\\
&(\operatorname{Ln}(x))^{2}+2 \operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(x)+(\operatorname{Ln}(2)-\operatorname{Ln}(3))(\operatorname{Ln}(2)+\operatorname{Ln}(3))=0 \\\\
&(\operatorname{Ln}(x))^{2}+2 \operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(x)+\operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(6)=0
\end{aligned}\]
\[\begin{aligned}
&\text { Let } y=\operatorname{Ln}(x)\\\\
&y^{2}+2 \operatorname{Ln}\left(\frac{2}{3}\right) y+\operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(6)=0\\\\
&\Delta=\left(2 \ln \left(\frac{2}{3}\right)\right)^{2}-4 \ln \left(\frac{2}{3}\right) \operatorname{Ln}(6)\\\\
&=4\left(\operatorname{Ln}\left(\frac{2}{3}\right)\right)^{2}-4 \operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(6)\\\\
&=4 \operatorname{Ln}\left(\frac{2}{3}\right)\left(\operatorname{Ln}\left(\frac{2}{3}\right)-\operatorname{Ln}(6)\right)\\\\
&=4 \operatorname{Ln}\left(\frac{2}{3}\right)(\operatorname{Ln}(2)-\operatorname{Ln}(3)-\operatorname{Ln}(3)-\ln (2))\\\\
&=4 \operatorname{Ln}\left(\frac{2}{3}\right)(-2) \operatorname{Ln}(3)\\\\
&=-8 \operatorname{Ln}\left(\frac{2}{3}\right) \operatorname{Ln}(3)
\end{aligned}\]
\[\begin{aligned}
=4 \operatorname{Ln}\left(\frac{3}{2}\right) & \operatorname{Ln}(9)>0 \\\\
y=\operatorname{Ln}(x) &=-\operatorname{Ln}\left(\frac{2}{3}\right) \pm \sqrt{\operatorname{Ln}\left(\frac{3}{2}\right) \operatorname{Ln}(9)} \\\\
&=\operatorname{Ln}\left(\frac{3}{2}\right) \pm \sqrt{\operatorname{Ln}\left(\frac{3}{2}\right) \operatorname{Ln}(9)}
\end{aligned}\]
We don’t want any sides to have a negative value, thus
\[\begin{aligned}
&\ln (x)=\operatorname{Ln}\left(\frac{3}{2}\right)+\sqrt{\operatorname{Ln}\left(\frac{3}{2}\right) \operatorname{Ln}(9)} \\\\
&x=e^{\ln \left(\frac{3}{2}\right)+{\sqrt{\operatorname{Ln}\left(\frac{3}{2}\right) \operatorname{Ln}(9)}}} \\\\
&x=\frac{3}{2} e^{\sqrt{\operatorname{Ln}\left(\frac{3}{2}\right) \operatorname{Ln}(9)}}
\end{aligned}\]
Home -> Solved problems -> Triangle problem, logarithms
Every problem you tackle makes you smarter.
↓ Scroll down for more maths problems↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?