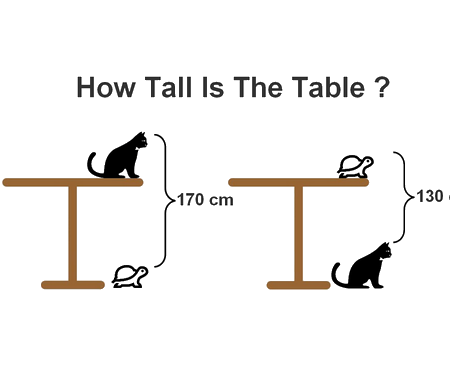
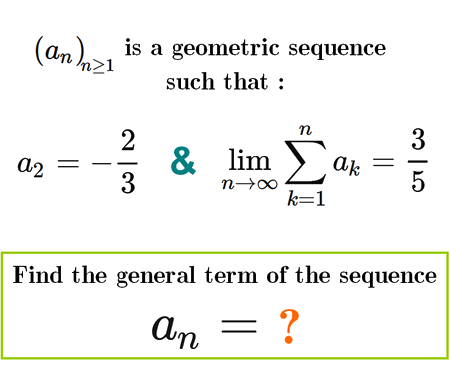
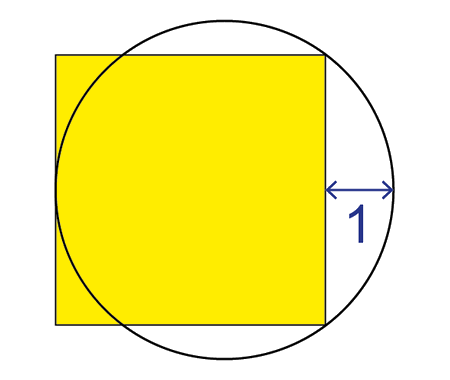
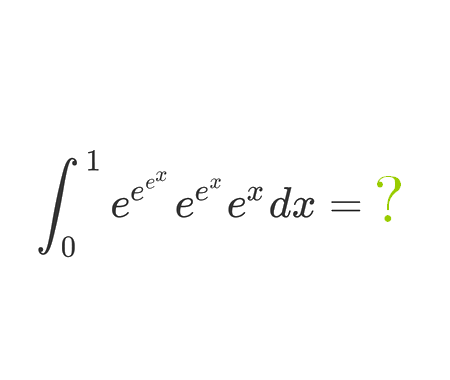Home -> Solved problems -> Volume of kiln
Volume of kiln

Solution
Let \(x>0\)
\[\begin{aligned}
3-\cosh x =0 \\\\
\cosh x =3 \\\\
x= \ln (3+2 \sqrt{2})
\end{aligned}\]
\[\begin{gathered}
v^{*}=\{(x, y, z): 0 \leqslant x \leqslant \ln (3+2 \sqrt{2}),\;\;\; 0 \leqslant y \leqslant 3-\cosh x ,\;\;\;
0 \leqslant z \leqslant 6\}
\end{gathered}\]
\[v=2 v^{*}\]
\[v=2 \iiint_{v} d v
\] \[=2 \int_{0}^{\operatorname{ln}(3+2 \sqrt{2})} d x \int_{0}^{3-\cosh x} d y \int_{0}^{6} d z
\] \[=2 \int_{0}^{\ln(3+2 \sqrt{2})} d x \int_{0}^{3-\cosh x}6\; d y
\] \[=\left.12 \int_{0}^{\ln\left(3+2\sqrt{2}\right)} y\right|_{0} ^{3-\cosh x} d x
\] \[=12 \int_{0}^{\left.(\ln3+2\sqrt{2}\right)}(3-\cosh x) d x
\] \[=12[3 x-\sinh x]_{0}^{\ln (3+2 \sqrt{2})}
\] \[=12[3 \ln (3+2 \sqrt{2})-\sinh (\ln (3+2 \sqrt{2}))]
\]
\[\cong 29,5178 \ldots
\]
Home -> Solved problems -> Volume of kiln
Every problem you tackle makes you smarter.
↓ Scroll down for more maths problems↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?