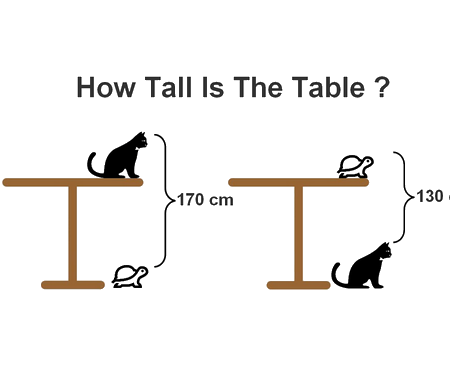
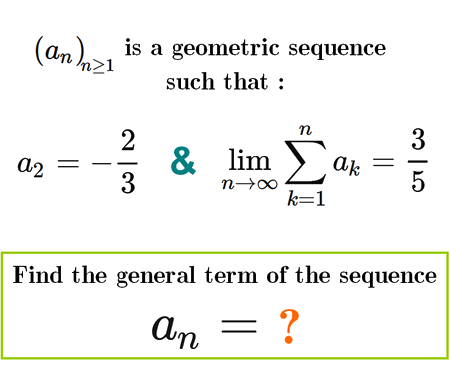
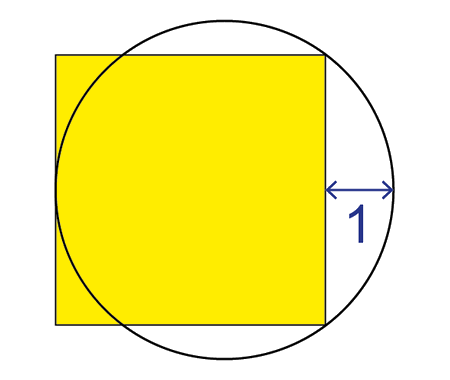
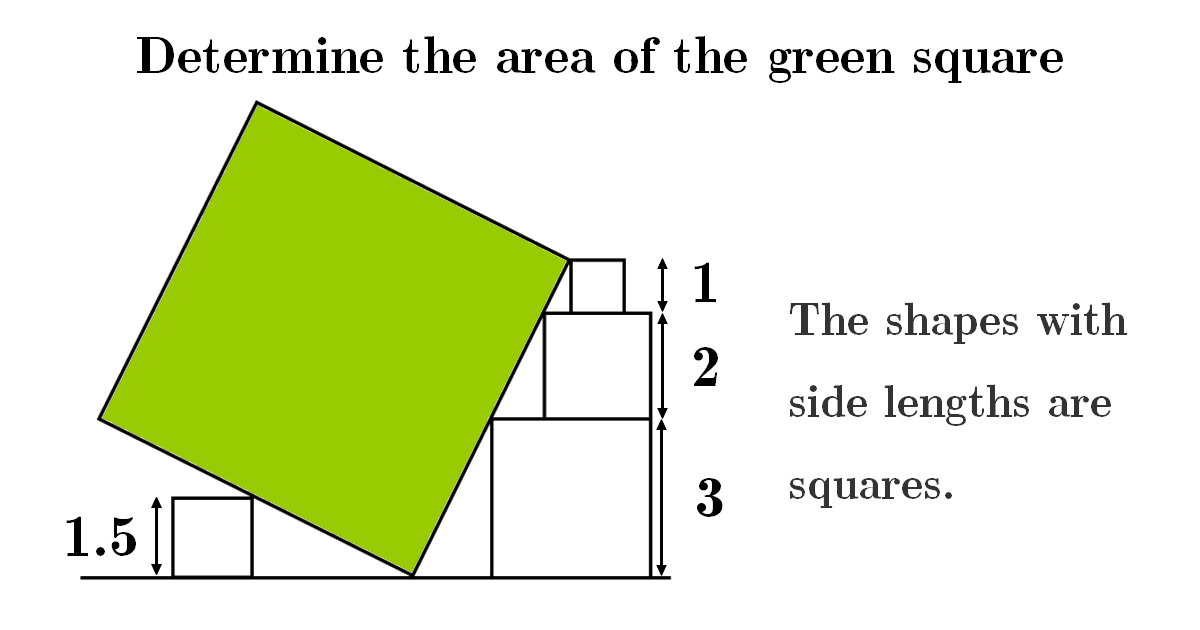
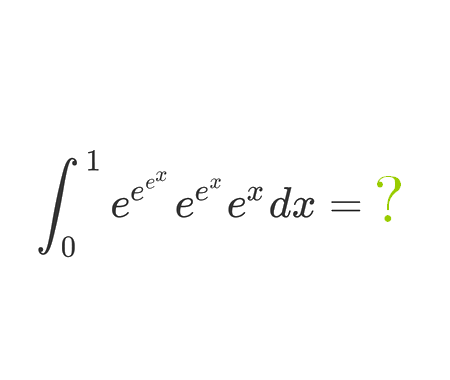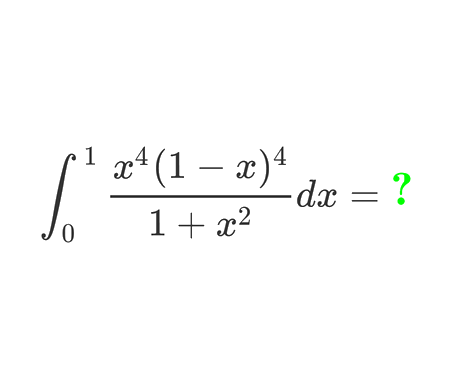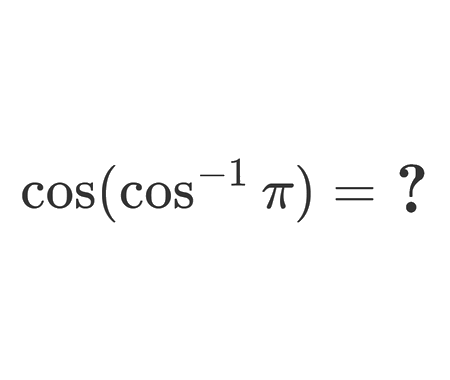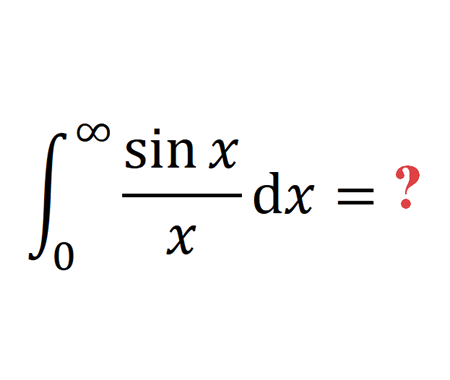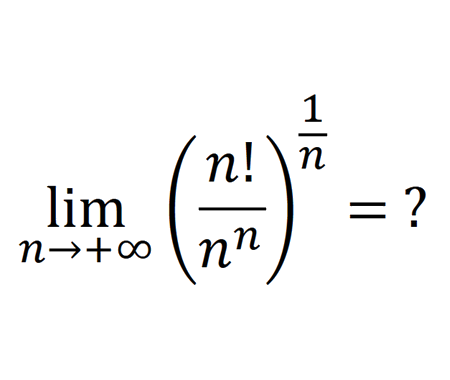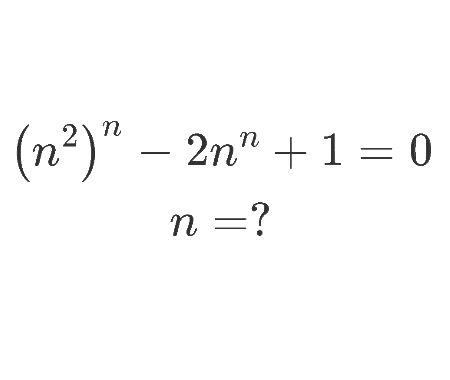Determine the area of the green square
Home -> Solved problems -> Determine the area of the green square
Solution
On the left side we have a square with side \(1.5\). On the right side we have three squares of sides \(1,\; 2\;\) and \(\; 3\)
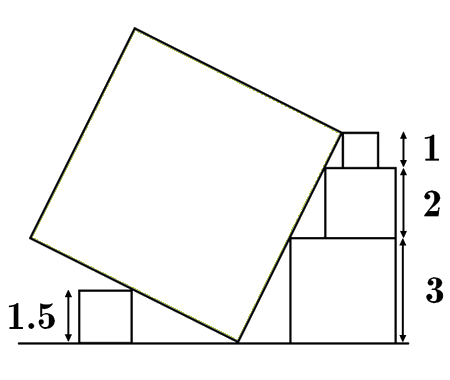
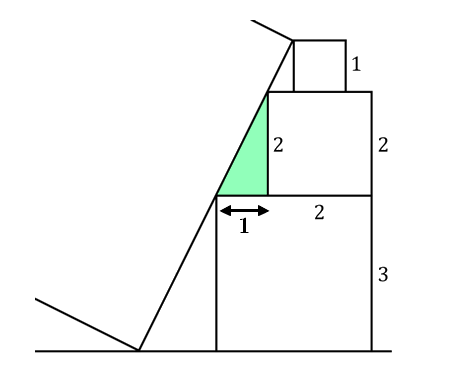
Using similarity, we get a vertical side which is twice the horizontal side.

If the square’s side is equal to \(x\)
, then its area is equal to \(x^{2}\). Using Pythagoras theorem in the right triangle we get:
\[x^{2}= 3^{2} + 6^{2}\]
\[=45\]
Therefore, the green square’s area is equal to \(45\).
Home -> Solved problems -> Determine the area of the green square
Every problem you tackle makes you smarter.
↓ Scroll down for more math problems↓
↓ ↓
↓ ↓
↓ ↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
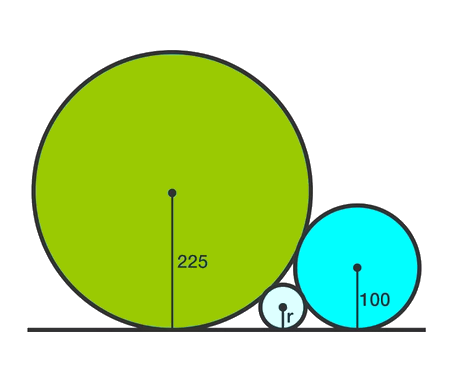
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
↓ ↓
↓ ↓
↓ ↓
↓ ↓
Home -> Solved problems -> Determine the area of the green square