Home -> Solved problems -> What is (-1)^pi equal to?
What is \((-1)^{\pi}\) equal to?
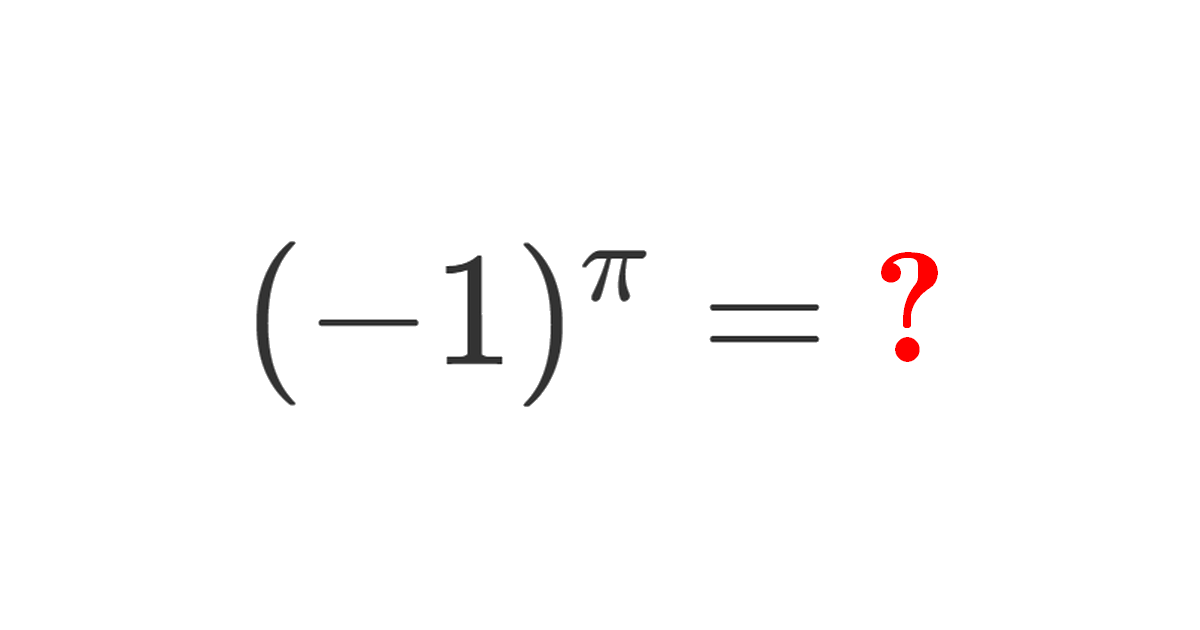
Solution
\[\begin{aligned}
&(-1)^{\pi}=? \\\\
&\begin{aligned}
e^{i \theta} &=\cos \theta+i \sin \theta \\\\
e^{i \pi} &=\cos \pi+i \sin \pi \\\\
&=-1
\end{aligned} \\\\
&\begin{aligned}
(-1)^{\pi} &=\left(e^{i \pi}\right)^{\pi} \\\\
&=e^{i \pi^{2}} \\\\
&=\cos \pi^{2}+i \sin \pi^{2} \\\\
& \simeq-0.903-(0.430) i
\end{aligned}
\end{aligned}\]
Home -> Solved problems -> What is (-1)^pi equal to?
Related Topics: Solved Problems
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
Home -> Solved problems -> What is (-1)^pi equal to?