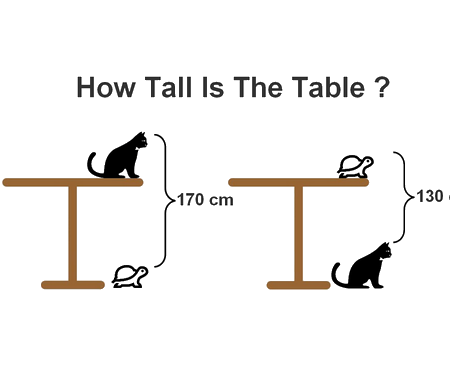
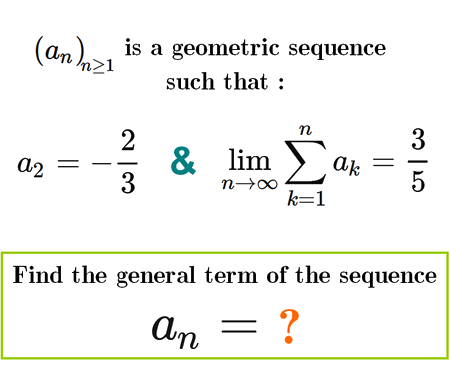
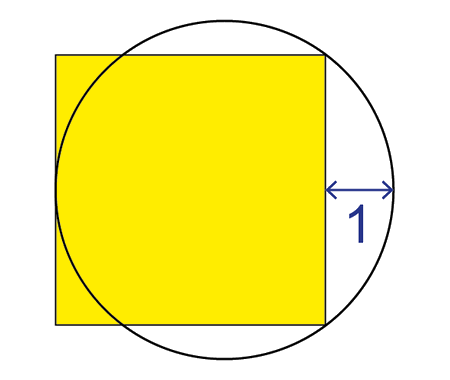
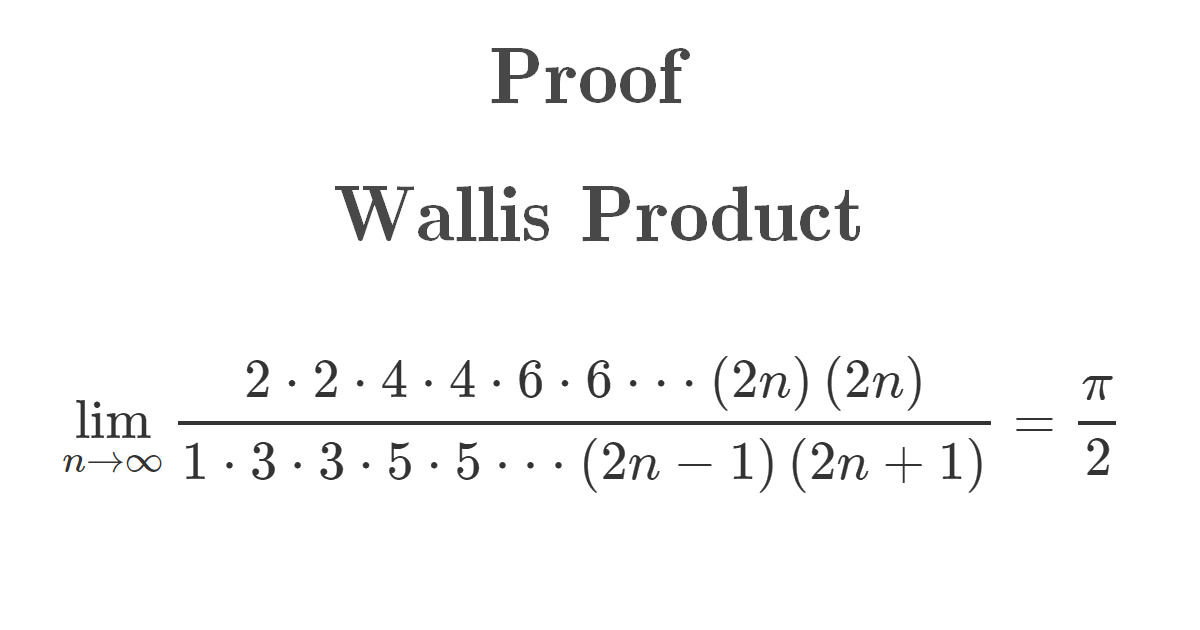
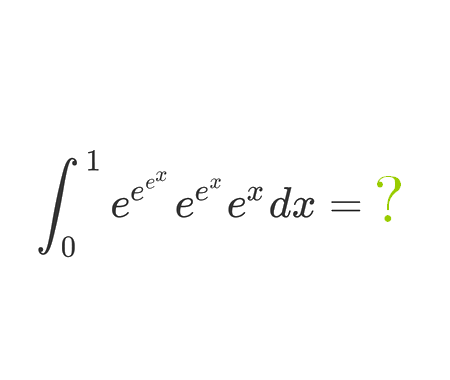Proof Wallis Product Using Integration
Home -> Solved problems -> Wallis product
Solution
Consider \(J_{n}=\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n}\text{d}x\) \[\] Integrating by parts with \(u\left(x\right)=\left(\cos\left(x\right)\right)^{n-1}\) and \(v’\left(x\right)=\cos\left(x\right)\) \[\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n}\text{d}x=\left(n-1\right)\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n-2}\left(\sin\left(x\right)\right)^{2}\text{d}x\] \[=\left(n-1\right)\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n-2}\left(1-\left(\cos\left(x\right)\right)^{2}\right)\text{d}x\] \[=\left(n-1\right)\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n-2}\text{d}x-\left(n-1\right)\int_{0}^{\frac{\pi}{2}} \left(\cos\left(x\right)\right)^{n}\text{d}x\] Gathering terms, we get \[nJ_{n}=\left(n-1\right)J_{n-2}\] We have \(J_{1}=1\) so recursively \(J_{3}=\frac{2}{3}\), \(J_{5}=\frac{2\cdot4}{3\cdot5}\) and inductively \[J_{2n+1}=\frac{2\cdot4\cdot\cdot\cdot\left(2n-2\right)\left(2n\right)}{1\cdot3\cdot\cdot\cdot\left(2n-1\right)\left(2n+1\right)}\] Likewise \(J_{2}=\frac{\pi}{2\cdot2}\), \(J_{4}=\frac{3\pi}{2\cdot4\cdot2}\), \(J_{6}=\frac{3\cdot5\cdot\pi}{2\cdot4\cdot6\cdot2}\) and inductively \[J_{2n}=\frac{3\cdot5\cdot\cdot\cdot\left(2n-3\right)\left(2n-1\right).\pi}{2\cdot4\cdot\cdot\cdot\left(2n-2\right)\left(2n\right)\cdot2}\] For \(0\leq x\leq\frac{\pi}{2}\) we have \(0\leq \cos\left(x\right)\leq1\) thus \[\left(\cos\left(x\right)\right)^{2n}\geq\left(\cos\left(x\right)\right)^{2n+1}\geq\left(\cos\left(x\right)\right)^{2n+2}\] Implying therefore that \[J_{2n}\geq J_{2n+1}\geq\ J_{2n+2}\] Then \[1\geq \frac{J_{2n+1}}{J_{2n}}\geq\frac{J_{2n+2}}{J_{2n}}=\frac{2n+1}{2n+2}\] Hence \[\lim_{n \rightarrow \infty}\frac{J_{2n+1}}{J_{2n}}=1\] Therefore \[\lim_{n \rightarrow \infty}\frac{2\cdot2\cdot4\cdot4\cdot6\cdot6\cdot\cdot\cdot\left(2n\right)\left(2n\right)}{1\cdot3\cdot3\cdot5\cdot5\cdot\cdot\cdot\left(2n-1\right)\left(2n+1\right)}\frac{2}{\pi}=1\] Or equivalently \[\color{black} {\lim_{n \rightarrow \infty}\frac{2\cdot2\cdot4\cdot4\cdot6\cdot6\cdot\cdot\cdot\left(2n\right)\left(2n\right)}{1\cdot3\cdot3\cdot5\cdot5\cdot\cdot\cdot\left(2n-1\right)\left(2n+1\right)}=\frac{\pi}{2}}\]
Home -> Solved problems -> Wallis product
Every problem you tackle makes you smarter.
↓ Scroll down for more maths problems↓
↓ ↓
↓ ↓
↓ ↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
↓ ↓
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
↓ ↓
↓ ↓
↓ ↓
↓ ↓
Home -> Solved problems -> Wallis product