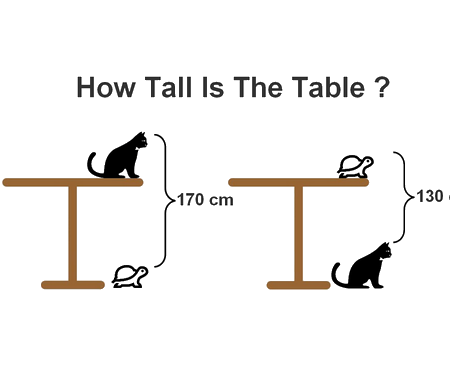
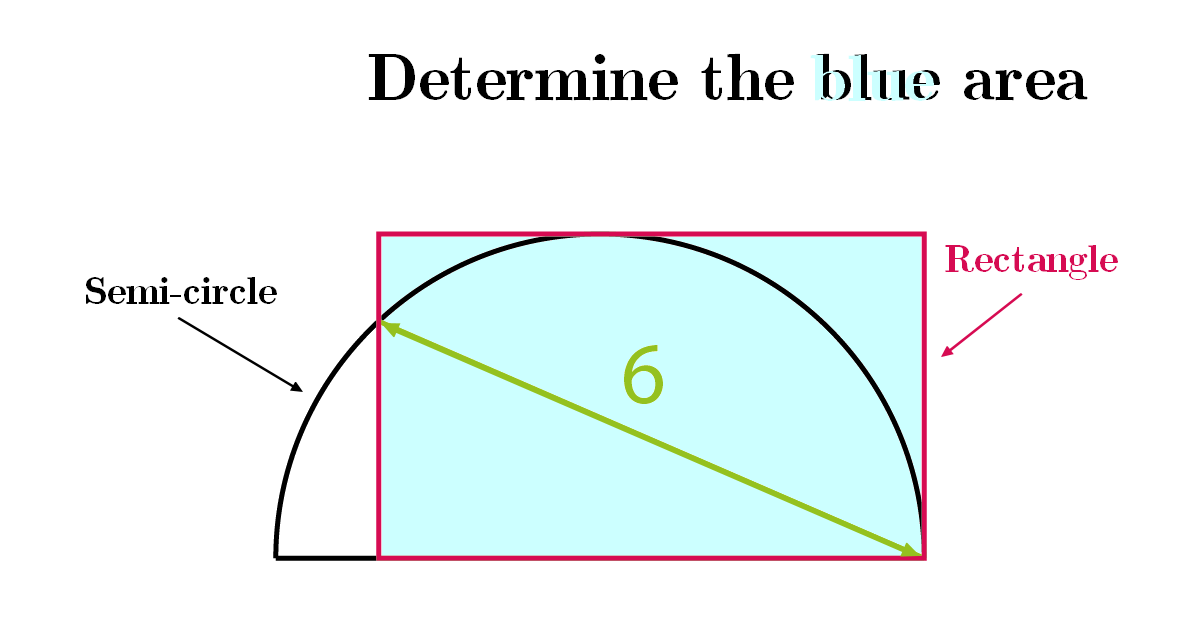
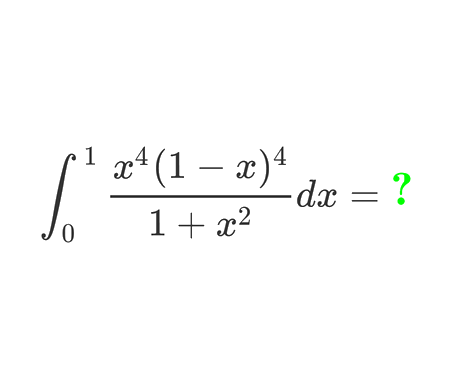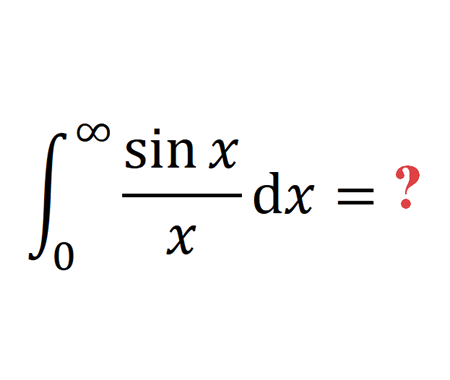Determine the rectangle's area (Semi-circle and rectangle)
Home -> Solved problems -> Calculate the rectangle’s area
Solution
At first, we add some sections to the semi-circle and the rectangle like it is shown in the next figure
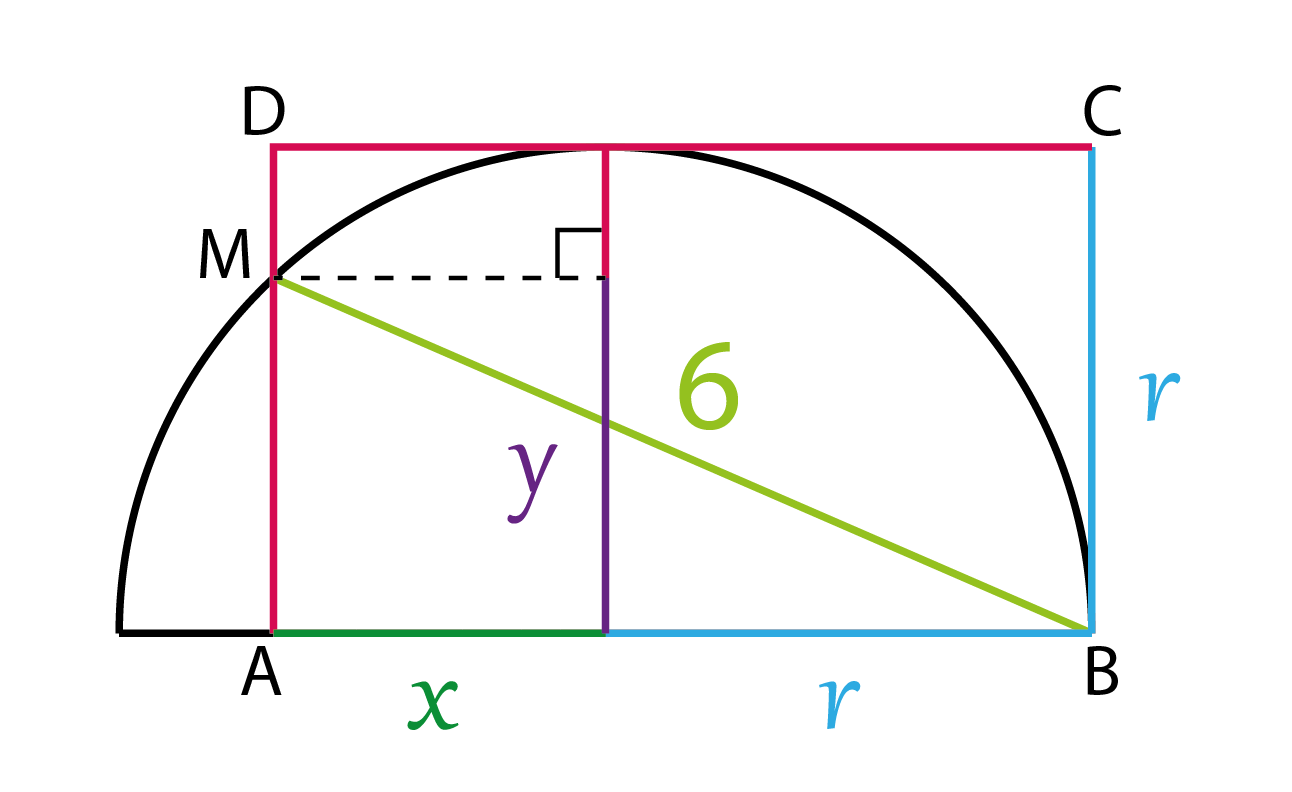
Using Pythagoras theorem in the triangle \(\triangle MAB\), we get \[\begin{array}{r}
(r+x)^{2}+y^{2}=6^{2} \\
=36 \\
r^{2}+2 r x+x^{2}+y^{2}=36
\end{array}\] \(x^{2}+y^{2}=r^{2}
\) : Circle equation at the point \(M
\), thus \[\begin{array}{r}
r^{2}+2 r x+r^{2}=36 \\
2r^{2}+2rx=36 \\
r^{2}+rx=18 \\
r(r+x)=18 \\
\Rightarrow BC\cdot AB=18
\end{array}\]
Home -> Solved problems -> Calculate the rectangle’s area
Every problem you tackle makes you smarter.
↓ Scroll down for more maths problems↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
Home -> Solved problems -> Calculate the rectangle’s area