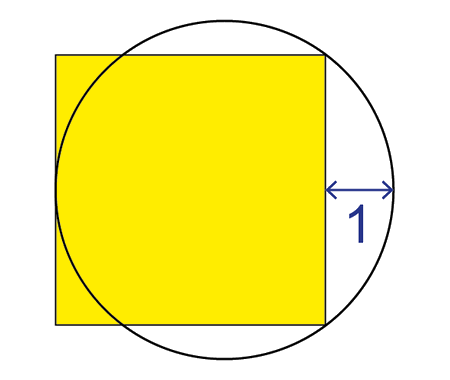
Home -> Solved problems -> What is the area of the square?
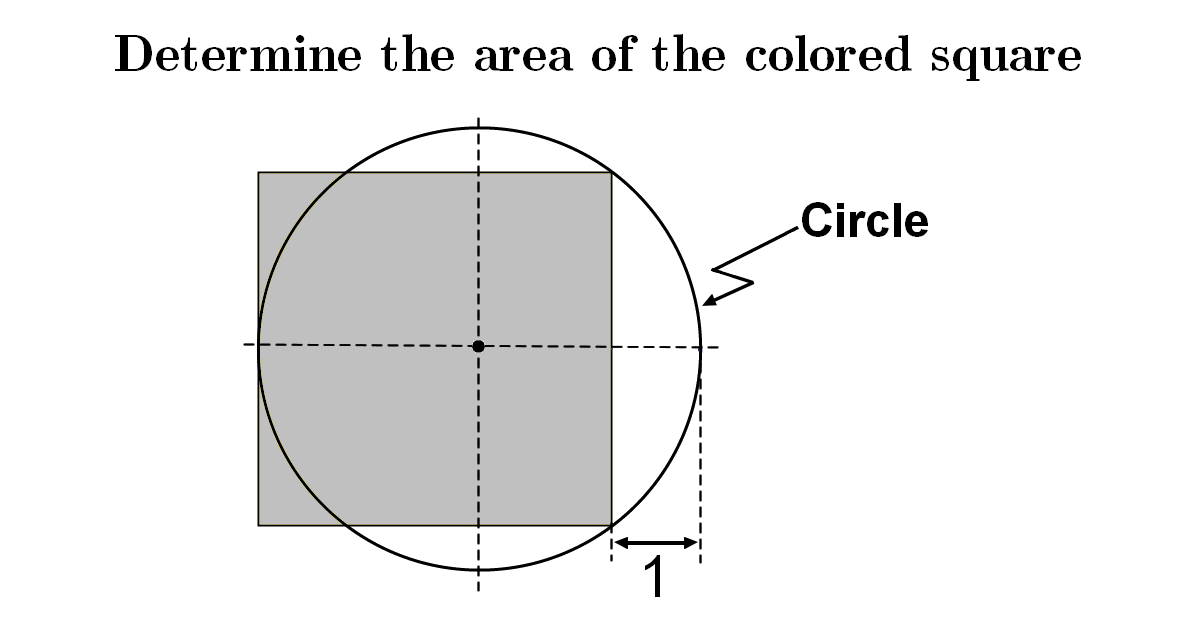
Solution
To start, let \(s\) be the side length of the square and \(r\) the radius of the circle. Let’s simplify the problem using the next figure.
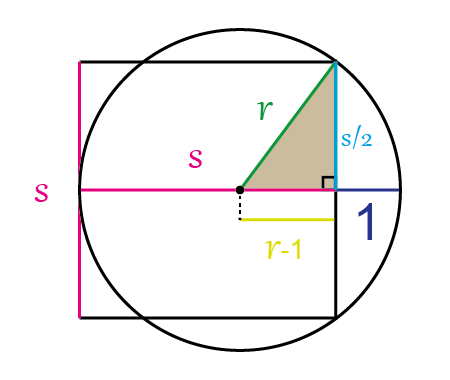
The square’s area is equal to \(s^{2}\), let’s find the side length of the square \(s\). Using the previous figure, we get
\[\begin{aligned}
&s+1=2 r\;\;\;\;\;\;\;(1)\\\\
&\text {Using Pythagoras theorem in the right grey triangle, we get}\\\\
&r^{2} =(r-1)^{2}+\left(\frac{s}{2}\right)^{2} \\\\
&r^{2} =r^{2}-2 r+1+\frac{s^{2}}{4} \\\\
&1-2 r+\frac{s^{2}}{4} =0 \\\\
&4-8 r+s^{2} =0\\\\
&\text {Using (1), we get}\\\\
&(2 r-1)^{2}-8 r+4=0 \\\\
& 4 r^{2}-4 r+1-8 r+4=0 \\\\
& 4 r^{2}-12 r+5=0 \\\\
& 4\left(r-\frac{1}{2}\right)\left(r-\frac{5}{2}\right)=0 \\\\
&r=\frac{1}{2} \quad Or \quad r=\frac{5}{2} \\\\
&r=\frac{s}{2}+\frac{1}{2}>\frac{1}{2} \\\\
\Rightarrow & r=\frac{5}{2} \\\\
\Rightarrow & \text { Square’s area }=s^{2}=(2 r-1)^{2}=16
\end{aligned}
\]
Share the solution :
Home -> Solved problems -> What is the area of the square?
Every problem you tackle makes you smarter.
↓ Scroll down for more maths problems↓
Prove that the function \(f(x)=\frac{x^{3}+2 x^{2}+3 x+4}{x}
\) has a curvilinear asymptote \(y=x^{2}+2 x+3\)
Why does the number \(98\) disappear when writing the decimal expansion of \(\frac{1}{9801}\) ?
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
Home -> Solved problems -> What is the area of the square?