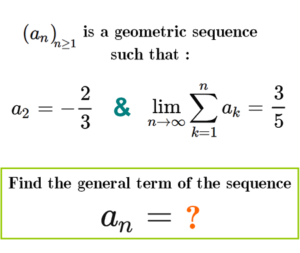
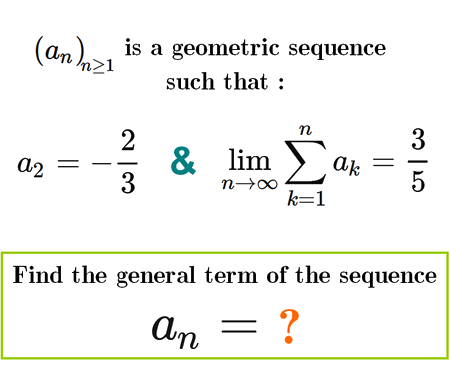
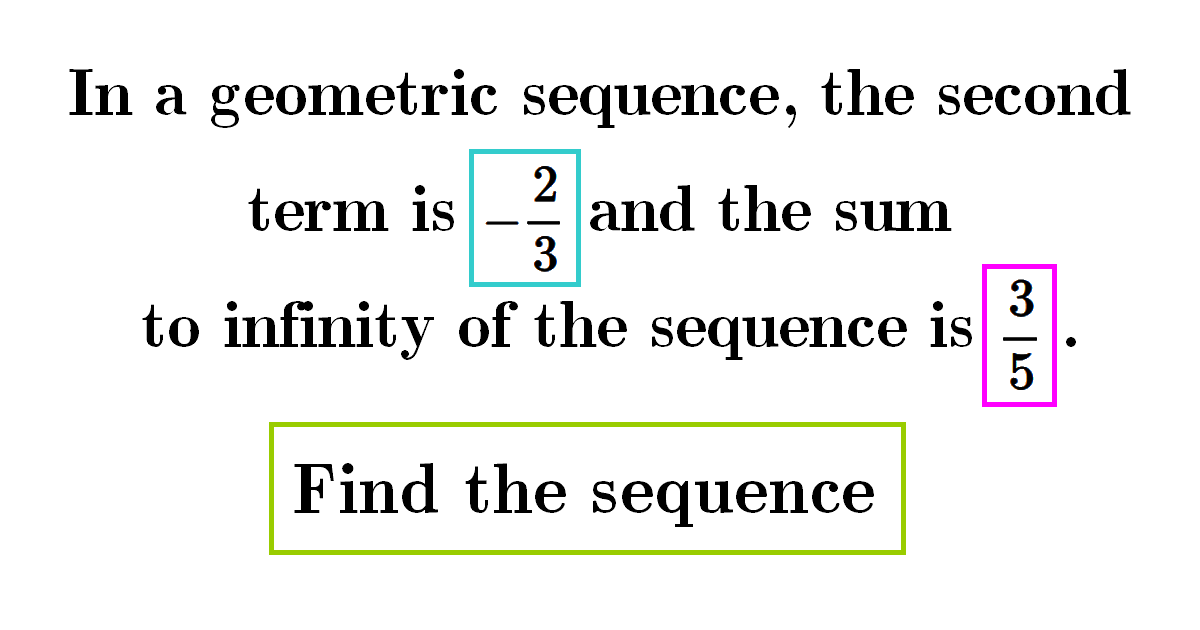
Home -> Solved problems -> Find the general term of the sequence
Solution
To start, we know that we are dealing with a geometric sequence, thus
\[a_{2}=r a_{1}=-\frac{2}{3}\;\;\;\;\;\;\;(1)\]
\(r\) is the common ratio.
An infinite geometric series converges if and only if \(|r|<1\), therefore
\[\begin{aligned}
\lim _{n \rightarrow\infty} \sum_{k=1}^{n} a_{k}&=\frac{a_{1}}{1-r} \\\\
\Rightarrow \frac{a_{1}}{1-r}&=\frac{3}{5}\;\;\;\;\;\;\;(2)
\end{aligned}\]
\((1)\) and \((2)\), gives
\[\begin{aligned}
&\frac{a_{1}}{1+\frac{2}{3 a_{1}}}=\frac{3}{5} \\\\
&\frac{a_{1}}{\frac{3 a_{1}+2}{3 a_{1}}}=\frac{3}{5} \\\\
&\frac{3 a_{1}^{2}}{3 a_{1}+2}=\frac{3}{5} \\\\
&3 a_{1}^{2}=\frac{9}{5} a_{1}+\frac{6}{5} \\\\
&3 a_{1}^{2}-\frac{9}{5} a_{1}-\frac{6}{5}=0 \\\\
&15 a_{1}^{2}-9 a_{1}-6=0 \\\\
&15\left(a_{1}-1\right)\left(a_{1}+\frac{6}{15}\right)=0 \\\\
&a_{1}=1\;\;\;\; or\;\;\;\; a_{1}=-\frac{6}{15}
\end{aligned}\]
Let’s discuss the found values
\[\begin{aligned}
&a_{1}=1\;\;\;\; gives \;\;\;\;r=-\frac{2}{3}\\\\
&a_{1}=-\frac{6}{15}\;\;\;\; gives \;\;\;\;r=\frac{5}{3}>1\;\;\;\;\text { not possible because }|r|<1\\\\
&\text { So we consider the results } a_{1}=1 \text { and } r=-\frac{2}{3}
\end{aligned}
\]
The \(n\)-th term of a geometric sequence with first term \(a_{1}\) and common ratio \(r\) is given by
\[\begin{aligned}
&a_{n}=a_{1} r^{n-1} \\\\
&a_{n}=\left(-\frac{2}{3}\right)^{n-1} \quad n \geqslant 1
\end{aligned}
\]
Like & Share the solution on Facebook :
Home -> Solved problems -> Find the general term of the sequence
Every problem you tackle makes you smarter.
↓ Scroll down for more math problems↓
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
Home -> Solved problems -> Find the general term of the sequence
Share the solution: Find the general term of the sequence