Home -> Solved problems -> Calculate the integral \int_{0}^{1}(-1)^{x} dx
Calculate the integral \(\int_{0}^{1}(-1)^{x} d x\)
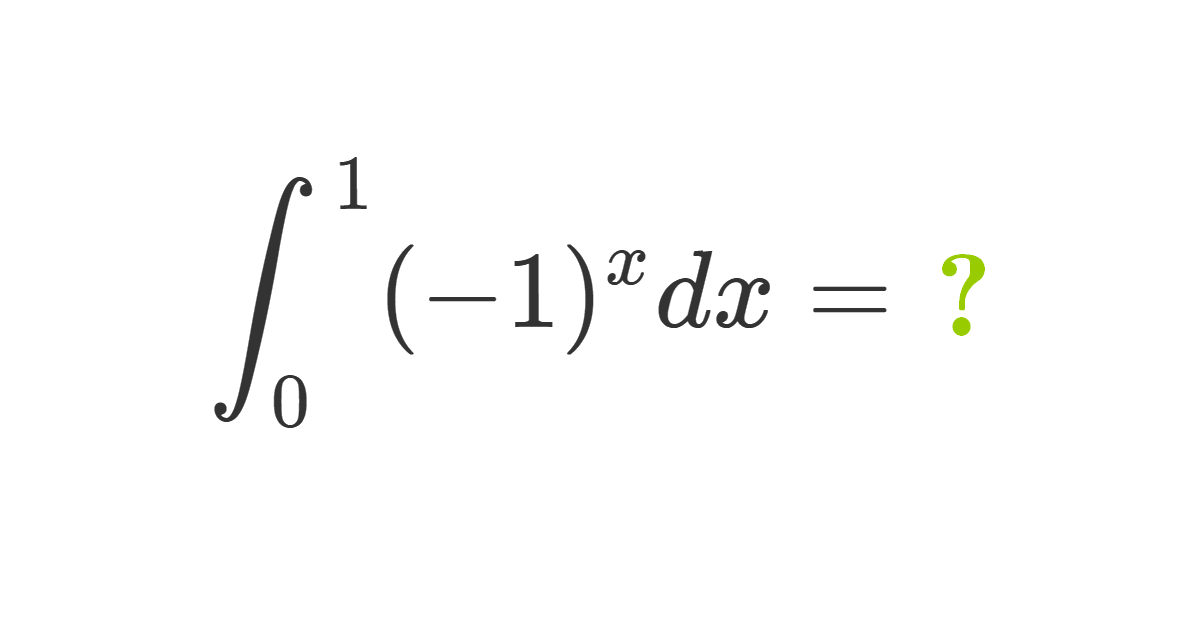
Solution
What is the integral from 0 to 1 of negative 1 raised to the power of
\(x\)
Let’s learn how to solve this problem
\[\begin{aligned}
e^{i \theta} &=\cos \theta+i \sin \theta \\\\
e^{i \pi} &=\cos \pi+i \sin \pi \\\\
&=-1
\end{aligned}\]
\[\begin{aligned}
(-1)^{x}&=\left(e^{i \pi}\right)^{x} \\\\
(-1)^{x}&=e^{i \pi x} \\\\
\text { for } &x>0\\\\
\int_{0}^{1}(-1)^{x} d x &=\int_{0}^{1} e^{i \pi x} d x \\\\
&=\left(\frac{e^{i \pi x}}{i \pi}\right)_{0}^{1} \\\\
&=\frac{e^{i \pi}}{i \pi}-\frac{1}{i \pi}\\\\
&=\frac{-1}{i \pi}-\frac{1}{i \pi} \\\\
&=\frac{-2}{i \pi}
\end{aligned}\]
Home -> Solved problems -> Calculate the integral \int_{0}^{1}(-1)^{x} dx
Every problem you tackle makes you smarter.
↓ Scroll down for more math problems↓
if we draw an infinite number of circles packed in a square using the method shown below, will the sum of circles areas approach the square's area?
Home -> Solved problems -> Calculate the integral \int_{0}^{1}(-1)^{x} dx
Share the solution: Calculate the integral \(\int_{0}^{1}(-1)^{x} d x\)